Explore our Research Centres
Delivering world class research
Research at the University of Newcastle is supported by a collaborative network of institutes and centres.
Filter centres
Major Centres
Hunter Medical Research Institute
The Hunter Medical Research Institute (HMRI) supports the Hunter's internationally recognised health and medical research, education and training. HMRI is a multidisciplinary partnership between the University of Newcastle and Hunter New England Local Health District and the community. Established in 1998, HMRI facilitates collaborations between researchers translating scientific advances into better clinical care, competitive commercial products and improved health care guidelines.
Newcastle Institute for Energy and Resources
At the Newcastle Institute for Energy and Resources (NIER), multidisciplinary teams are driving productivity and sustainability gains through applied research focused on innovation that is delivering transformational solutions in sectors of national significance.
University Research Centres
Centre for Critical Minerals and Urban Mining
The Centre for Critical Minerals and Urban Mining is concerned with the science and engineering of particulate systems relevant to industries of national significance, such as in the mineral resources area. We seek to develop faster and more efficient separation technologies, and technologies for the manufacture, storage, and transport of particles.
Centre for Digestive Health and Gastroenterology
The Centre for Digestive Health and Gastroenterology brings together multi-disciplinary team which aims to effectively diagnose and treat common functional gut disorders (dyspepsia and IBS), neuromuscular diseases of the gut and eosinophil-driven chronic inflammatory gastrointestinal conditions through technologically advanced research which has identified novel pathophysiological mechanisms.
Centre for Geotechnical Science and Engineering
The Centre for Geotechnical Science and Engineering develops new models and innovative computational methods for predicting the behaviour of geomaterials, metals and composites. Advanced computational methods, coupled with laboratory and field testing are key tools in this pursuit.
Centre for Innovative Energy Technologies
The Centre for Innovative Energy Technologies conducts cutting edge research on emerging energy technologies, with particular focus on the abatement of greenhouse gases, and clean and sustainable energy production.
Centre for Prevention, Implementation and Population Health
Centre for Prevention, Implementation and Population Health uses intervention and service delivery to promote healthy behaviours and good quality healthcare across communities.
Centre for Women's Health Research
The Centre for Women's Health Research brings together academics and clinicians working in women’s health research and consumers with a passion for women’s health to research the factors that affect the health and wellbeing of women across the life course.
Australian Research Council (ARC) centres and hubs
Centre of Excellence for Enabling Eco-Efficient Beneficiation of Minerals
This Centre will transform the minerals industry, establishing a new generation of research leaders to support the innovation needed in creating a green economy for future generations.
National Health and Medical Research Council (NHMRC) centres
Australia Centre for Cannabinoid Clinical and Research Excellence (ACRE)
The Australia Centre for Cannabinoid Clinical and Research Excellence (ACRE) is Australia's first federally funded research centre in medicinal cannabinoids. It brings together researchers from more than 10 institutions to build capacity in medicinal cannabis research and provide a national response to current challenges.
This NHMRC-funded centre will develop a well-governed collaborative strategy to generate world-class research in medicinal cannabis. It will also be pivotal in establishing Australia as a world leader in appropriately balanced implementation of medicinal cannabinoid uptake in specialist and primary health care settings.
Centre of Research Excellence for Venom and Antivenom
The Centre of Research Excellence in Translational Venom and Antivenom Research is a collaboration of five Australian universities and two Sri Lankan universities and brings together experts in the fields of venom and antivenom research. The Centre will focus on snake envenoming and the effectiveness and safety of antivenom. Central to improving the treatment of snake bite is the early identification of envenoming as there is increasing evidence that early administration of antivenom is the key to good outcomes. Key to achieving this aim is the development of a cheap, quick bedside test for envenoming and this will be one of the main objectives of the Centre.
Centre of Research Excellence in Asthma Treatable Traits
The NHMRC Centre of Research Excellence in Asthma Treatable Traits (CREATT) aims to revolutionise the management of chronic airway diseases by testing and implementing a new paradigm for personalised medicine.
Building on outcomes of the Centre of Excellence in Severe Asthma and its ground-breaking toolkit, CREATT is a consortium of world-renowned clinicians, researchers and respiratory organisations who are generating new knowledge to support the treatable traits approach and ensure translation into practice.
Centre of Research in Digestive Health
The NHMRC Centre for Research Excellence in Digestive Health aims to improve quality of life for patients with unexplained chronic gastrointestinal disorders. It brings together clinical researchers from universities, hospitals and research institutions across Australia and beyond.
The centre supports research, training, multidisciplinary collaboration, and translation of findings to enhance our understanding, identification and management of chronic digestive diseases.
National Centre of Implementation Science
The centre brings together a multi-disciplinary team of emerging and established researchers to generate evidence that helps fast-track the adoption of interventions for chronic disease prevention.
Partner organisations for this NHMRC Centre for Research Excellence include the University of Sydney, Ottawa Hospital Research Institute, Monash University, Central Queensland University and Neuroscience Research Australia.
Cooperative Research Centres (CRC)
Hosted Cooperative Research Centres (CRC)
Soil CRC
The Cooperative Research Centre for High Performance Soils (Soil CRC) is bringing together scientists, industry and farmers to find practical solutions for Australia’s underperforming soils. The Soil CRC aims to enable farmers to increase their productivity and profitability by providing them with knowledge and tools to improve the performance of their soils. It is the biggest collaborative soil research effort in Australia’s history, with 39 Participants that contribute to the Soil CRC through both cash and in-kind contributions.
Partner Cooperative Research Centres (CRC)
HILT CRC
This Cooperative Research Centre aims to help Australia’s heavy industry sector compete in the low-carbon global economy by developing solutions to help reduce its greenhouse gas emissions.
The sector processes minerals such as iron, steel, and aluminium which support the production of critical materials for day-to-day life and makes a significant contribution to Australia’s industrial sovereignty. The HILT CRC will focus on overcoming barriers to the sector’s low-carbon transition.
iMove CRC
The Australian Transport Cooperative Research Centre, known as the iMOVE CRC, is a national transport research and development centre dedicated to providing a coordinated approach to Australian transport research.
It is a consortium of more than 40 industry, government and research partners engaged in a 10-year effort to improve Australia’s transport systems. It aims to deliver solutions that help reduce road congestion, fuel use, emissions, accidents and fatalities. It also works to improve freight co-ordination, productivity, international competitiveness, and overall lifestyle.
MinEx CRC
The MinEx Cooperative Research Centre is working to develop more productive, safer and more environmentally friendly drilling methods for critical deposits that lie beneath sand, soil and sediment. It is also investing the development of new exploration tools and new ways to deploy those tools, to support longer-term sustainability and safety in the sector.
SmartCrete CRC
Concrete is the second most used material on earth after water and is a fundamental element of our built environment. The SmartCrete Cooperative Research Centre provides a nationally coordinated and collaborative platform for research and development in Australia.
It brings together leading industry partners, research institutions, agencies and associations to focus on innovation in engineered solutions, asset management and sustainability.
Multi-Institutional Research Centres
Australian Gastrointestinal Research Alliance
The Australian Gastrointestinal Research Alliance (AGIRA), led by Laureate Professor Nick Talley and Professors Marjorie Walker (Newcastle) and Gerald Holtmann (Brisbane) is a coalition of researchers in gastrointestinal (GI) disease who have expertise in clinical medicine and gastroenterology, mucosal immunology, translational science, pathology, imaging, psychology, and epidemiology in aspects of GI disease in Australia.
Central Coast Research Institute for Integrated Care (CCRI)
The Central Coast Research Institute (CCRI) is a joint venture of the University of Newcastle and Central Coast Local Health District. The CCRI aims to deliver pioneering research relevant to improving the health and wellbeing of the Central Coast community and beyond, focusing on the design, implementation and evaluation of new models of person-centred integrated care.
Clinical Nutrition Research Centre
Professor Peter Howe from the School of Biomedical Sciences and Pharmacy is Director of the Clinical Nutrition Research Centre (CNRC). It offers Australia's foremost expertise in the design and conduct of human intervention trials to evaluate cardiovascular, metabolic, anti-inflammatory and mental health benefits of functional nutrients, foods and nutraceuticals.
College centres
Advanced Cyber Security Research Centre
The overall aim of the Advanced Cyber Security Research Centre (ACSRC) is to achieve fundamental advances in the theory, design and management of models and techniques that would enable secure and dependable computing information systems and services.
An important characteristic of ACSRC is its research capabilities and expertise covering both theory and practice in addressing the challenges that arise in achieving secure systems in a heterogeneous mobile distributed environment involving distributed systems, wireless, broadband and peer to peer networks, mobile devices and distributed information services.
African Research, Engagement and Partnerships
The focus of the Centre for African Research, Engagement and Partnerships (CARE-P) is to lead and further enhance the global research capacity, academic excellence, social reputation and humanity of the University of Newcastle through engaging in sustainable and high impact research, teaching and professional consultancy with world class African universities, educational providers, governments and non-government partners.
Brain and Mental Health Research
The Centre for Brain and Mental Health Research (CBMHR) is focused on increasing our understanding of the brain and mind across the lifespan, in the absence and presence of disease. The Centre hosts three platforms for research: Preclinical Neurobiology, Psychological Processes, and Mental and Physical Health.
Building Science Technology and Sustainability
Building Science, Technology and Sustainability research provides internationally recognised research and knowledge in sustainable infrastructure and building design, construction and management through a multi-inter-trans-disciplinary approach
Bulk Solids and Particulate Technologies
The Centre for Bulk Solids and Particulate Technologies (CBSPT) is actively involved in both fundamental and applied research on a range of problems associated with bulk solids and particulate technology. Research areas include storage, flow, processing and transportation of bulk solids. CBSPT also provides specialist courses for industry through its professional development programs.
Centre for Advanced Energy Integration
The Centre for Advanced Energy Integration is focused on research that integrates new energy technologies and systems related to energy storage, grid systems, energy generation and alternative energy sources.
Centre for Construction Safety and Well-being
The Centre for Construction Safety and Well-being (CCSW) is a collaboration between the University of Newcastle and construction company Hansen Yuncken, located within the university's School of Architecture and Built Environment.
Centre for Drug Repurposing and Medicines Research
The Centre for Drug Repurposing & Medicines Research is committed to improve quality, safety, efficacy and timelines for bringing the most effective drug therapies to patients.
Centre for Rehab Innovations
The University of Newcastle’s Centre for Rehab Innovations (CRI) is a Centre of Excellence in technology-enabled health care innovations and rehabilitation research.
Computer Assisted Research Mathematics and its Applications
The Centre for Computer Assisted Research Mathematics and its Applications (CARMA) explores the development of techniques and tools for computer-assisted discovery and data-mining, including mathematical visualisation.
Family Action Centre
The Family Action Centre (FAC) is a research, teaching and practice centre focused on families and their communities. The Centre has a 30 year track record of innovative, dynamic and enduring work that aims to strengthen family and community wellbeing. The assets and diverse resources of families and communities underpin vibrant, cohesive and resilient societies. The FAC is committed to addressing the factors that affect the health, wellbeing, social and educational paths of families, particularly for those challenged by increasingly complex vulnerabilities.
Global Centre for Environmental Remediation (GCER)
The Global Centre for Environmental Remediation (GCER) aims to safeguard people's social, economic and physical health and wellbeing by developing innovative, cost-effective and sustainable technologies and solutions that reduce the impact of pollutants on the environment.
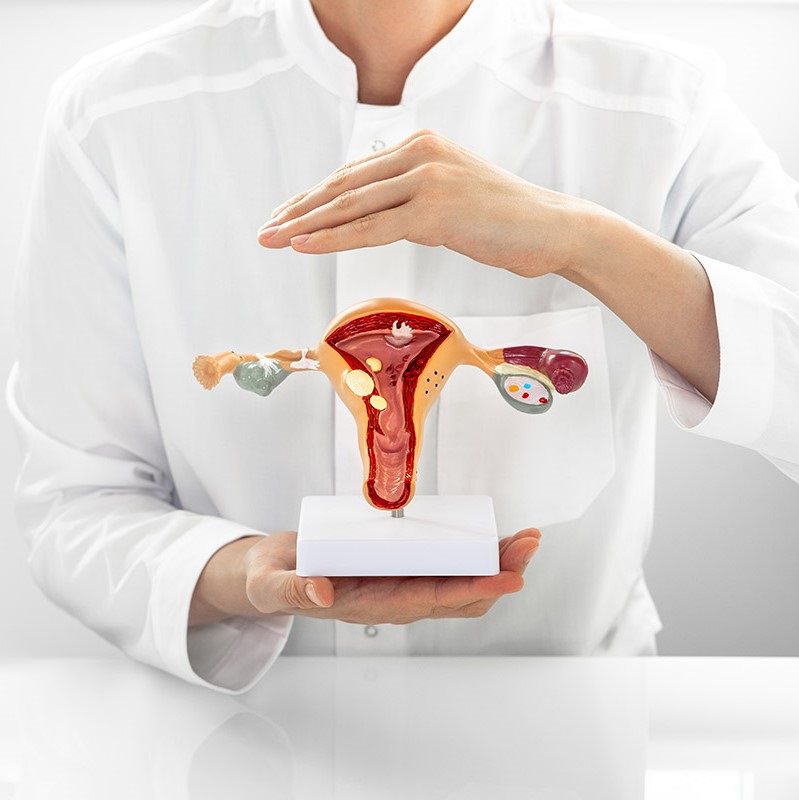
Global Centre for Gynaecological Diseases
The Global Centre for Gynaecological Diseases is a world-leading research centre focused on improving the gynaecological health of women and aims to pioneer a personalised approach to the prevention, detection, and treatment of gynaecological diseases.
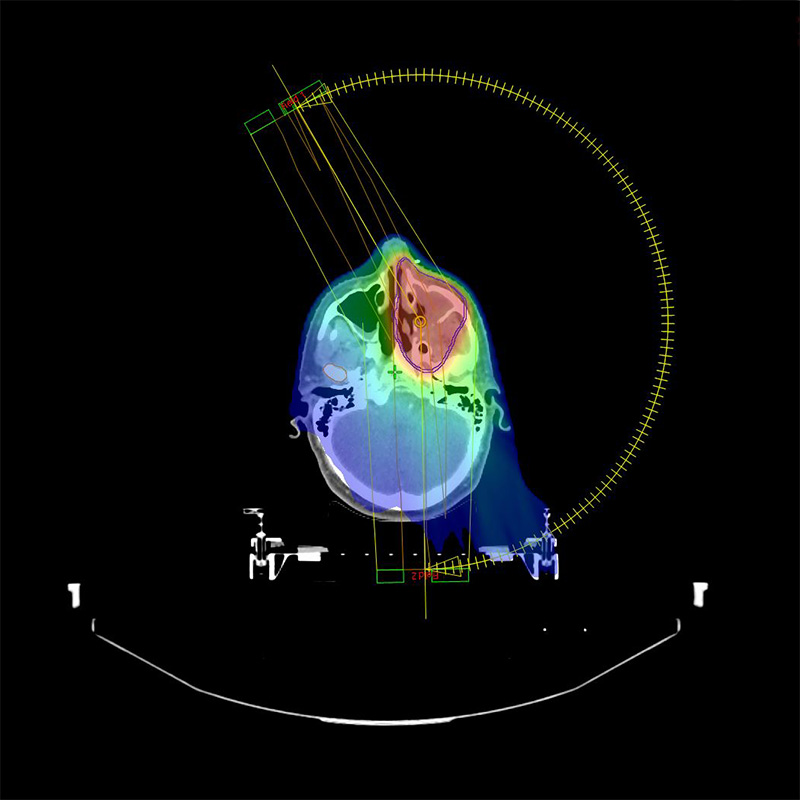
Global Centre for Research and Training in Radiation Oncology
The Global Centre is the first of its kind. With the support of industry partners and collaborators, the centre delivers education, research and training to domestic and international clinicians and students.
Global Innovative Centre for Advanced Nanomaterials (GICAN)
Innovation in materials science and research to develop advanced technologies and solutions for the global energy, environment and health sectors.
Health Professional Education
The Centre for Health Professional Education is committed to engaging in a systematic and rigorous program of research that will examine the effectiveness of curricula interventions as well as the impact of health professional education on patient outcomes.
Health Services
The Health Services Research and Innovation Centre (HSRIC) aims to improve the design and management health services with particular emphasis interdisciplinary approaches to addressing critical challenges.
HunterWiSE
HunterWiSE is an initiative that establishes mentorship avenues for women in STEM throughout the Hunter region; promoting positive collaboration and sharing of experiences.
Indigenous Knowledge Research Network
The Indigenous Knowledge research network is led by Indigenous academics, researchers, and Community knowledge-holders with the aim to respond to the needs and interests that support and promote the unique perspectives, knowledge and contributions of Aboriginal and Torres Strait Islander people.
Infrastructure Performance and Reliability
The Centre for Infrastructure Performance and Reliability (CIPAR) is at the international forefront in assessing the reliability, safety, durability and management of buildings, bridges, pipelines, aviation, and other infrastructure systems. The pioneering research at CIPAR includes risk and reliability assessment, corrosion of infrastructure, and structural masonry.
International Collaborative Centre for Carbon Futures
An international research collaboration network developing innovative technologies for a sustainable carbon economy.
Ironmaking Materials Research
The Centre for Ironmaking Materials Research (CIMR) is a partnership between NIER and BHP Billiton, aimed at defining the link between fundamental iron ore and metallurgical coal properties and their performance in the ironmaking process.
Mark Hughes Foundation Centre for Brain Cancer Research
The Mark Hughes Foundation Centre for Brain Cancer Research is committed to finding a cure and improving the lives of those affected by brain cancer. We aim to advance brain cancer research and achieve the greatest impact for brain cancer patients and their families.
Mass and Thermal Transport in Engineering Materials
The Centre for Mass and Thermal Transport in Engineering Materials has a very strong research capability in the theory and simulation of mass and thermal transport in a wide variety of materials ranging from disordered and ordered multicomponent alloys to cation-mixed oxides, from metal/ceramic composites to fast ion conductors.
Medical Engineering Research
The Centre has a strong program of medical engineering research spanning applications including medical signal processing, image analysis, virtual reality in medical settings, artificial organs, precision mechatronics, personalised medicine and intelligent implants.
Multiphase Processes
The Centre for Multiphase Processes conducts research in the science and technology of fine particles and bubbles. Multiphase processes, which involve more than one phase of matter, form the basis for all Australian process industries including food, energy, agricultural, production and processing.
Organic Electronics
The Centre for Organic Electronics is focused on the scientific challenges in the development of organic photovoltaics for the next generation of environmentally friendly energy sources, photonics and biosensors.
Plant Science
The Centre for Plant Science is focused on the physiological and molecular mechanisms associated with the regulation of nutrient transport in higher plants.
Purai - Global Indigenous and Diaspora Research
Purai is an exciting research initiative to integrate global and transnational analytical perspectives and frameworks with research on Indigenous and diaspora studies, and other related topics of race and society.
Resources Health and Safety
The Centre for Resources Health and Safety draws on the multidisciplinary expertise of University researchers spanning disciplines including workplace health and safety, psychology, psychiatry, respiratory studies and environmental impacts, to meet sector demand in this area and facilitate industry and government collaborations for the benefit of communities in resource intensive regions.
Rural and Remote Mental Health
The Centre for Rural and Remote Mental Health (CRRMH) has a long-standing history of providing evidence-based translational research and expert advice on mental health for people living in rural and remote Australia. Throughout its 20 years of operation, CRRMH improved the provision of mental health services through the identification of and response to the needs of rural and remote communities. In particular, this includes the development of appropriate service models for rural mental health care, providing professional development opportunities for clinical staff and offering education and training.
Secure and Reliable Communications
The Centre for Secure and Reliable Communications contributes to developing telecommunication networks that are robust to eavesdropping attacks, interference, and noise.
Space Physics
The Centre for Space Physics examines space weather to better understand its impact on billions of dollars’ worth of spacecraft and related infrastructure.
Urban and Regional Studies
The Centre for Urban and Regional Studies engages in research focused on the complex political, economic, social, cultural and environmental processes and relations transforming cities and regions.
Water Security and Environmental Sustainability
Water controls Australia’s well-being, environmental health and economy. The Centre for Water Security and Environmental Sustainability (CWSES) is a strategic initiative that builds upon research strengths of ERA 5 groups. It brings together key researchers in Civil, Surveying and Environmental Engineering and Earth Sciences.
Water, Climate and Land
The Centre for Water, Climate and Land focuses on understanding and dealing with the impacts of climate variability and change in the Asia-Pacific region. Of particular interest are hydrological extremes and how these may change in the future.
College groups
College of Engineering, Science and Environment
Coastal and Marine Science
The Coastal and Marine Science (CaMS) Research Group is a cross-disciplinary team dedicated to improving the health and sustainability of our coastal and marine environments and the societies that depend upon them. The research spans across urban catchment environments, coastal waterways and lakes to the ocean; extending geographically from tropical to temperate locales.
Cognitive Psychology
Cognitive Psychology takes a broad and integrative approach to understanding mental processes. We have particular strength in mathematical modeling and pursue research that integrates behavioural and neuroscience measures.
Conservation Science
The Conservation Science Research Group at The University of Newcastle consists of a team of internationally recognised experts in biodiversity conservation with project experience in the natural environment and biodiversity; environmental monitoring, restoration ecology, behavioural ecology and cognition, instrumentation and analysis; marine pollution impacts; and sustainable adaptation in agriculture and forestry.
Disaster and Development
To advance knowledge and understanding on disaster resilience and sustainable development.
Distributed Computing
The Distributed Computing Research Group (DCRG) is focused on investigating distributed and network computing concepts and implementations.
Environment and Climate
The Environment and Climate Research Group conduct internationally significant research in hydrology, climatology, palaeoclimatology and landscape modelling. We develop the science and translate it into information or tools that are useful for industry stakeholders or policy makers.
Environmental Engineering
Engineers are developing new innovative computer and other models to provide methods of assessment of environmental impacts and management of disturbed ecosystems.
Environmental Plastic Innovation Cluster
EPIC conducts cutting-edge research on the risk assessment of legacy plastics and plastic-alternate products. Our vision is to ensure that plastic-alternative materials are environmentally sustainable and safe, leading to better planetary health.
Environmental Water Science
Our research group study water’s importance to ecosystems and humans, its use and management as a resource, and its modification through contamination and pollution.
Exercise and Sport Science
Research within Exercise and Sport Science is interdisciplinary and diverse. Our research covers topics ranging from improving elite sports performance to the positive effects of exercise for both general and clinical populations.
Fluid Mechanics and Turbulence
The Fluid Mechanics and Turbulence Research Group is applying an advanced understanding of the physics of turbulence to developments in turbulence computation, control and management.
Food Science
The University of Newcastle’s Food Science Research Group combines active research scientists involved in food, plant and marine science, human nutrition and agribusiness, delivering quality research and research education opportunities of relevance to the sector.
Health and Clinical Psychology
The Health and Clinical Psychology Research Group conducts fundamental and applied research into factors associated with psychological wellbeing and physical health across the lifespan.
Hydrogen Technology and Materials
The renewable Technology and Materials Group unites applied and theoretical expertise alike of several academics from the School of Engineering at the University of Newcastle. More specifically, our expertise comprises photocatalytic hydrogen generation, design of next-generation fuel cell stacks, infrastructure durability, advanced materials science, and manufacturing.
i3Lab – Interaction, Interfaces, Invention
Human-Computer Interaction research that explores how advanced interactive technologies are applied to real-world problems. The i3 Lab addresses industry-relevant problems where significant value can be gained by the invention of new solutions related to the complex interactions that occur between the humans, computers and data.
Interdisciplinary Machine Learning
The Interdisciplinary Machine Learning Research Group (IMLRG) investigates aspects of machine learning, pattern recognition and data analysis in theory, experiments and applications. Particular emphasis is placed on interdisciplinary projects.
Knowledge Engineering Research Team
The Knowledge Engineering Research Team (KERT) brings together academics at the University of Newcastle who have research interests in knowledge engineering, smart knowledge management systems, multi-agent agents, knowledge-based embedded systems and augmented reality.
Marine Natural Products and Chemical Ecology
Marine Natural Products Chemistry is the study of the structure and chemistry of compounds produced by marine plants and animals such as algae and sponges, and their role in nature.
Medical Physics
The Medical Physics Research Group comprises physicists based within the Radiation Oncology Department, Calvary Mater Newcastle Hospital. We are a member of the Centre for Information Based Medicine, University of Newcastle and Hunter Medical Research Institute. We are closely aligned with the Prostate Cancer Trials Group and the Trans-Tasman Radiation Oncology Group (TROG) which organise and conduct clinical trials throughout Australasia.
Metabolic Research
The Metabolic Research Group comprises academics studying metabolism at the cellular, tissue and body levels. Research focuses on measuring alterations to homeostasis at the cellular level in the case of bacteria and the whole body in relation to responding to external stimuli.
Nanomaterials Research Group
The Nanomaterials Research Group is using advanced materials for application in energy storage, sensing and bioimaging.
Newcastle Robotics Laboratory
The Newcastle Robotics Laboratory is an interdisciplinary research and training initiative of several robotics related research teams from different research areas including applied mathematics, architecture, computer science, control, electrical engineering, neuroscience, mechatronics and software engineering.
Precision Mechatronics Lab
A multidisciplinary group of electrical engineers, mechanical engineers and physicists developing new mechatronic and robotic technologies for fabrication, imaging, and health care
Surface and Nanoscience
Our primary research focus is on the modelling and analysis of the structure of the surface of solids, from the surface atomic layer through to the bulk.
Tectonics and Earth Resources
Our research examines the lithospheric processes that form the physical environment in which we live. This research is achieved within a plate-tectonic framework that focuses on the formation and dispersal of continents.
Telecommunications Networks
The Telecommunications Networks Research Group is engaged in research and development across a range of new telecommunications technology, in areas such as 4G wireless communication systems, channel coding techniques, Smart Grid Technology, and machine-to-machine communications.
Water Resources Engineering
The Water Resources Engineering Research Group are developing innovative computer models to provide methods of assessment of environmental impacts and management of disturbed ecosystems.
Wind Energy
The Wind Energy Research Group focuses on the continued design and development of highly-efficient, low-cost small wind turbines.
College of Health, Medicine and Wellbeing
Adult Lifestyle Incentives for Vitality and Energy
ALIVE is a cross-disciplinary collaboration among our University community that supports health professionals to assist their clients with weight management through lifestyle changes.
Australian Centre for Evidence Based Nutrition and Dietetics
ACEBaND participates in the University of Newcastle evidence-based synthesis group; which is an affiliated centre of the University of Western Sydney in collaboration with the Joanna Briggs Institute (JBI). It is the only centre with an exclusive focus on nutrition internationally.
Chlamydia Research
The Chlamydia Research Group (CRG) is a major research group in the Discipline of Immunology and Microbiology. The group is funded by research grants from the NHMRC, the ARC, the HRC (NZ) and has substantial industry funding and is also part of the HMRI VIVA research group.
Clinical Epidemiology and Biostatistics
The Centre for Clinical Epidemiology and Biostatistics (CCEB) provides national and international education programs in public health for health professionals and the promotion and delivery of trans-disciplinary research into health and health care.
Clinical Pharmacology and Toxicology
The Discipline of Clinical Pharmacology and Toxicology is a multidisciplinary group of medicines experts spanning the scientific, clinical and social science aspects of medicines development, clinical trials regulation, pricing, clinical use of medicines and pharmaceutical policy.
Clinical Radiation Research
In Radiation Oncology, one patient's care is managed by many: radiation oncologists (doctors), medical physics specialists, radiation therapists and nurses. Administration staff manage the complex task of fitting in so many people to receive their treatment and follow-up, and Clinical Trials Coordinators make sure that when study participants agree to take part in a trial, they know what to expect.
Clinical Toxicology
The Clinical Toxicology Research Group focuses on improving understanding of poisoning and envenoming in patients and undertaking studies to determine the effectiveness of antidotes and antivenoms in treatment of these conditions.
Digital Mental Health Research Group
Led by Professor Rhonda Wilson and involving international and multidisciplinary networks of researchers and collaborating organisations, the Digital Mental Health Research Group is helping to reimagine the way technologies are used to enhance mental health care in Australia and worldwide.
Education and Nursing Research in Child Health
The Centre for Education and Nursing Research in Child Health's aim is to support and encourage nurses working in child and family health, neonatal, paediatric and adolescent health (hereafter termed child health nursing/nurses) to undertake research, ensure nursing practice in these specialty areas is evidence based and build nurses' post graduate education and professional development.
Medical Imaging and Radiation Therapy
The Medical Imaging and Radiation Therapy Research group focuses on research into the use of both ionising and non-ionising radiation in the diagnosis and treatment of disease.
Mental Health Nursing Research and Practice Development
The program of research operating from the Nursing Research and Practice Development Unit (NRPDU) locates research into mental health nursing within a broad framework of mental health services research. The main focus of the program is to assess the effectiveness of nursing led psychological and psychosocial interventions using qualitative and/or quantitative research methods.
Midwifery
The Midwifery Research Group is lead by Dr Virginia Skinner who has worked as a Research Project Officer for Associate Professor Martin Veysey for the previous five years on two studies, one assessing cardiovascular health in an elderly population and the other assessing the relationship between bitter taste, folate intake and the development of bowel polyps before commencing work at the University of Newcastle.
Mothers and Babies
The Mothers and Babies Research Centre is a multidisciplinary group working on maternal, foetal and neonatal health problems.
Nutraceuticals Research
The area of Nutraceuticals has long been the focus of the research group headed by Professor Manohar Garg. Nutraceuticals, dietary supplements and functional foods have been the research focus of the many successful research higher degree students and postdoctoral fellows. This group brings together a number of academic staff members and their research personnel from the Faculty of Health and Medicine, Faculty of Science & IT and Faculty of Education & Arts under one umbrella.
Professional Issues and Acute Care
Researchers involved in Professional Issues and Acute Care are focused on making a difference in the delivery of health services and for health professionals in the clinical environment, often using a multidisciplinary approach.
Speech Pathology
The speech pathology discipline is distinctive in its diversity and focus on the impact of communication disorders and disabilities in everyday life, as well as on key stakeholders supporting them.
Youth Outreach for Diabetes
Young people with type 1 diabetes are transferred from comprehensive outreach paediatric services to adult services at around 18 years of age. Many rural adult services have few resources to specifically support them, and the culture is more focused on compliance and long term complications. Young people are expected to have competent self management skills, which is often not the case as there are limited transition programs within Hunter New England Health. This issue is important as they are life longer users of the health care system.
College of Human and Social Futures
Sorry, no institutes or centres match your current selection, please adjust your filters.
PhD scholarships and opportunities
Find out more
The University of Newcastle acknowledges the traditional custodians of the lands within our footprint areas: Awabakal, Darkinjung, Biripai, Worimi, Wonnarua, and Eora Nations. We also pay respect to the wisdom of our Elders past and present.












![[ext] Australia Centre for Cannabinoid Clinical and Research Excellence](https://www.newcastle.edu.au/__data/assets/image/0009/865179/acre.jpg)

![[ext] Centre of Research Excellence in Asthma Treatable Traits](https://www.newcastle.edu.au/__data/assets/image/0008/865178/center-of-excellence-treatable-traits-logo.jpg)
![[ext] Centre for Research Excellence in Digestive Health](https://www.newcastle.edu.au/__data/assets/image/0006/865176/cre-digestive-health-logo.jpg)
![[ext] NCOIS](https://www.newcastle.edu.au/__data/assets/image/0005/870116/NCOIS-stackedlogo-fullcolour-onWhite-Small-.jpg)